Next: Control Structures and programming Up: Gröbner basis and Syzygy Previous: Computing Gröbner basis in Contents
Let ![]() be a ring and
be a ring and
![]() be elements of
be elements of ![]() .
The left
.
The left ![]() -module
-module
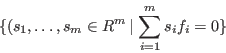
%% syz.sm1
[ (x,y) ring_of_differential_operators
[[(Dx) 1 (Dy) 1]] weight_vector
0
] define_ring
[ (x Dx + y Dy).
(Dx^2 + Dy^2).
] /ff set
ff { [[(h). (1).]] replace homogenize} map /ff2 set
[ff2 [(needBack) (needSyz)]] groebner /ans set ;
(Syzygies are ...) message
ans 2 get ::
The 0-th element of ans is the Gröbner basis.
The 1st element of ans is the transformation matrix from the input
to the Gröbner basis.
The 2nd element of ans is a set of generators of the syzygies
of the input.